Mathematics, often regarded as the universal language of science, is a profound testament to human curiosity and ingenuity. It encompasses more than just numbers and equations, embodying the relentless human quest to understand the intricacies of the world we inhabit. From its humble origins in the simple act of counting stones to its present-day involvement in deciphering the mysterious world of quantum physics, mathematics has evolved into an indispensable tool that has shaped the course of human history and continues to underpin the foundations of our modern world.
This post delves into the genesis of mathematics, tracing its journey from humble beginnings to complex quantum equations. By revisiting the milestones of mathematical development, we aim to appreciate the enduring significance of this discipline and how it has profoundly impacted our lives.
Early Mathematical Beginnings
The origins of mathematics can be traced back to prehistoric times when our ancestors began to count and quantify the world around them. Simple stone counting systems, where individual objects were represented by corresponding stones, marked the initial steps of mathematical thought.
The Role of Ancient Civilizations in Early Mathematics
Ancient civilizations played a crucial role in shaping the foundations of mathematics. [1] They recognized the practical and essential need for mathematical concepts in their daily lives, which led to significant developments. Here’s a closer look at the contributions of key ancient civilizations:
| Civilization | Contributions to Mathematics |
| Sumerians |
|
| Egyptians |
|
| Babylonians |
|
Sumerians
The Sumerians, one of the earliest civilizations in Mesopotamia, are credited with creating the first known writing system, cuneiform. This system allowed them to record numerical information and develop basic arithmetic. They used a base-60 system for calculations, which is still evident in our timekeeping and angle measurement systems (360 degrees in a circle, 60 minutes in an hour).
Egyptians
The ancient Egyptian empire is renowned for its architectural and engineering feats, including the construction of the pyramids. To achieve such remarkable structures, the Egyptians developed geometry and methods for surveying and measuring land. They also created practical systems for recording numbers and fractions, which were essential for taxation, engineering projects, and trade.
Babylonians
The Babylonians, residents of ancient Mesopotamia, are notable for their contributions to mathematics, particularly in algebra. They developed the earliest known mathematical text, the Plimpton 322 tablet, which contained lists of Pythagorean triples (integer solutions to the Pythagorean theorem). This tablet shows advanced mathematical thinking, and their understanding of algebraic relationships was ahead of its time.
These early civilizations laid the groundwork for concepts like arithmetic, geometry, and algebra. Ancient mathematics still influences modern scientific and technological advancements, making these civilizations critical to the genesis of mathematics.
Classical Greek Mathematics
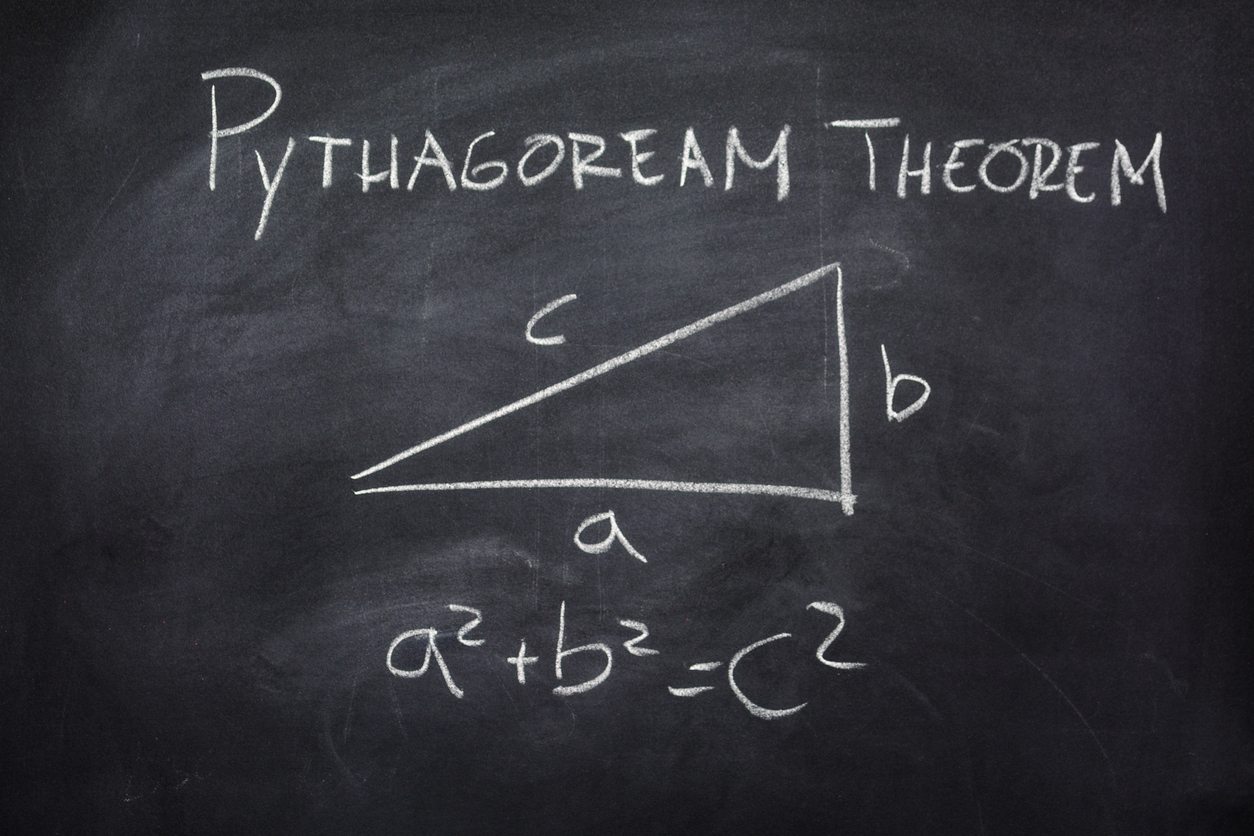
Ancient Greek Mathematics stands as a cornerstone in the history of mathematical development. The works of famous ancient Greek mathematicians like Pythagoras, Archimedes, and Euclid continue to influence mathematics even today. [2]
| Mathematician | Contributions to Mathematics |
| Pythagoras | Pythagorean theorem and number theory |
| Euclid | “Elements,” systematized geometry |
| Archimedes | Calculus, geometry, and principle of buoyancy |
Pythagoras
Pythagoras and his followers explored the relationships between numbers. He significantly advanced the understanding of geometry and numbers with the Pythagorean theorem which explains the relationship between the sides of a right triangle. His mathematical insights extended to music and led to the idea of mathematical proofs.
Euclid
Euclid’s “Elements” remains one of the most influential mathematical works ever written. This comprehensive compilation of knowledge covered geometry, number theory, and proofs, establishing rigorous principles for mathematical reasoning. He created a systematic framework for geometry and introduced the concept of proof, a fundamental principle in mathematics.
Archimedes
This Greek polymath made groundbreaking discoveries in geometry, calculus, and number theory. His work on the measurement of the circle and the method of exhaustion foreshadowed integral calculus, while his understanding of levers and buoyancy became foundational in engineering.
Besides contributing to the theory of mathematics, the ancient Greek mathematicians also set the stage for the development of mathematics as a formal science.
Mathematics in the Islamic Golden Age
During the Islamic Golden Age, spanning roughly from the 8th to the 13th century, scholars in the Islamic world played a pivotal role in preserving and advancing mathematical knowledge. [3]
| Mathematician | Contributions |
| Muhammad al-Khwarizmi | Pioneered algebra, introduced equations. |
| Al-Biruni | Advanced trigonometry and algebra. Calculated Earth’s radius. |
| Al-Kindi | Systematization of number theory and work on arithmetic. |
| Omar Khayyam | Solved cubic equations and studied algebra. |
| Nasir al-Din al-Tusi | Developed trigonometry and trigonometric functions. |
Preservation of Knowledge
Islamic scholars played a crucial role in translating and preserving classical Greek and Roman mathematical texts, preventing their loss during turbulent times in Europe. This preservation was instrumental in the revival of mathematical studies in the Western world later on.
Introduction of Algebra
One of the most significant contributions from this era was the development of algebra. The Persian mathematician Al-Khwarizmi wrote a foundational book “Al-Kitab al-Mukhtasar fi Hisab al-Jabr wal-Muqabala” (The Compendious Book on Calculation by Completion and Balancing) on the subject. His work laid the groundwork for symbolic algebra, paving the way for solving complex equations and making connections between arithmetic and geometry. The term “al-jabr” from the book’s title eventually gave algebra its name.
The Concept of Zero
Islamic mathematicians also introduced the revolutionary concept of zero, a fundamental numerical idea that was notably absent in many other mathematical traditions. This numeral, derived from the Arabic word “sifr,” significantly enhanced mathematical calculations.
Islamic mathematicians made pioneering advances in various mathematical disciplines, including arithmetic, geometry, and trigonometry, adding new dimensions to mathematics.
Renaissance and Modern Mathematicians
The European Renaissance era marked a transition from the mathematical ideas preserved and developed in the Islamic world and classical antiquity to a more modern approach. Prominent mathematicians of this era include:
René Descartes
Descartes was a French mathematician and philosopher. He introduced analytical geometry, merging algebra and geometry. [4] His development of a coordinate system paved the way for visualizing mathematical relationships on graphs, becoming a fundamental tool for contemporary mathematics and science. This innovation laid the foundation for analytic geometry, enabling the description of geometric shapes using algebraic equations.
Isaac Newton
Newton’s contributions to mathematics were profound as calculus revolutionized mathematics. [5] This new branch of mathematics provided methods to study change and motion, making it indispensable in fields such as physics and engineering.
Gottfried Wilhelm Leibniz
Leibniz was a German polymath who independently developed calculus at around the same time as Newton. His notation and approach to calculus have also significantly influenced modern mathematics. [6] Leibniz is known for introducing the integral sign (∫) and the use of the dy/dx notation for derivatives.
These Renaissance and modern mathematicians truly transformed mathematics. It became an essential tool for understanding and explaining the natural world.
The Age of Calculus and Differential Equations
This period witnessed the development of calculus which marked a crucial turning point in mathematical history. It opened the door to the intricate study of differential equations, having widespread applications across science and engineering.
The Birth of Calculus
In the late 17th century, English physicist and mathematician Isaac Newton and German polymath Gottfried Leibniz simultaneously introduced the world to calculus.
Newton’s Perspective
Newton used his calculus, known as the “method of fluxions.” He devised calculus as a tool to study change and motion. Newton’s Laws of Motion and the Law of Universal Gravitation, which are cornerstones of classical physics, were formulated using calculus.
Leibniz’s Approach
German polymath Gottfried Wilhelm Leibniz developed calculus independently and introduced the world to the concept of differentiation and integration. His notation system included symbols for differentiation (dy/dx) and integration (∫), making mathematical expression significantly more accessible.
The Role of Differential Equations
Calculus unveiled the utility of differential equations, which represent relationships involving rates of change. These equations emerged as a critical tool in the study of phenomena across various scientific disciplines. [7]
| Field | Role of Differential Equations |
| Physics | Describe motion, behavior of particles, and physical laws. |
| Engineering | Design, analyze, and optimize systems, circuits, and structures. |
| Biology | Model population dynamics, disease spread, and chemical reactions. |
| Chemistry | Express chemical reactions, reaction rates, and molecular interactions. |
| Environmental Science | Predict environmental changes, such as climate, pollution, and ecosystem dynamics. |
The Birth of Modern Algebra and Number Theory
The development of modern algebra saw significant contributions from renowned mathematicians such as Leonhard Euler and Carl Friedrich Gauss.
Euler’s Functions
The Swiss mathematician Leonhard Euler, famous for his contributions to various mathematical fields, played a significant role in developing algebraic concepts. [8] He introduced the notion of mathematical functions and notation, making algebra more systematic and structured.
Gauss’ Modular Arithmetic
Carl Friedrich Gauss made substantial contributions to algebra and number theory. [9] He developed modular arithmetic, which is essential in cryptography, and proved the fundamental theorem of algebra, demonstrating that every polynomial equation has a solution.
The Number Theory
Number theory, a branch of mathematics that deals with properties and relationships of numbers, found its importance in cryptography. This mathematical discipline helps create secure encryption methods by exploring the properties of prime numbers and their application in cryptographic algorithms.
Mathematics in the Age of Computers
As we venture into the modern era, it’s impossible to discuss mathematics without acknowledging the profound influence of computers. The advent of computing technology has revolutionized mathematical research and its practical applications.
| Aspect | Impact |
| Speed and Efficiency | Computers have dramatically accelerated mathematical calculations and problem-solving. Co |
| Exploration of Complex Problems | Computers enable mathematicians to tackle intricate and challenging mathematical problems that were previously beyond reach. |
| Numerical Simulations | Computational mathematics leverages computers to conduct numerical simulations that model real-world phenomena. Th |
| Data Analysis and Big Data | Mathematical techniques and algorithms enable data scientists to extract valuable insights from these data troves. |
| Interdisciplinary Collaboration | Computers facilitate collaboration between mathematicians and experts in other fields. |
Mathematics in the Age of Computers
The introduction of computers has opened up new horizons in mathematical research. Complex calculations and simulations that were once infeasible have now become routine. Mathematicians employ computational techniques to explore intricate problems in various fields, from physics to economics.
Computational Mathematics
Computational mathematics focuses on developing algorithms and numerical methods for solving mathematical problems. Computers play a pivotal role in computational mathematics, making it possible to tackle complex issues in various scientific and engineering domains.
Computers in Cryptography
Cryptography, the science of secure communication, has experienced a significant transformation with the integration of computer technology. Cryptographers employ advanced mathematical algorithms and computational techniques to create unbreakable codes and encryption methods.
Quantum Mathematics and Beyond
Mathematics plays a pivotal role in the intricate realm of quantum mechanics. This branch of physics explores the behavior of particles at the quantum level, where classical physics fails to provide adequate explanations.
Quantum mathematics is essential in understanding the behavior of subatomic particles. It deals with complex mathematical equations and theories that describe the behavior of particles at the quantum level. These equations are fundamental in quantum mechanics and form the basis for many of its widely used principles.
For instance, quantum computing utilizes these to perform computations that would be infeasible for classical computers. Similarly, quantum algorithms rely on advanced mathematical concepts to achieve exponential speedup.
Conclusion
The evolution of mathematics has been a remarkable journey, from ancient counting stones to the complex quantum equations of the modern age. As a universal language mathematics has continuously adapted and expanded to unravel the mysteries of the universe. It has helped shape the course of science, technology, and our understanding of reality. From the timeless theorems of ancient Greece to the intricate quantum calculations of today, mathematics remains an indispensable tool for unlocking the secrets of the cosmos. As we peer into the future, mathematics will undoubtedly continue to guide us through uncharted territories, opening new frontiers of knowledge and discovery.