World War II stands as one of the most significant conflicts in history, marked by an intricate web of strategies, alliances, and technological advancements. In the midst of this chaos, codebreaking emerged as a clandestine art in which mathematical acumen became the linchpin to deciphering encrypted messages. The unrelenting demand for intelligence, coupled with the intricacies of wartime ciphers, posed an unprecedented challenge.
This post delves into the mathematical odyssey that unfolded during World War II. It showcases the remarkable work of codebreakers who harnessed mathematical principles to unlock secrets that often held the balance between victory and defeat.
Early Codebreaking Efforts
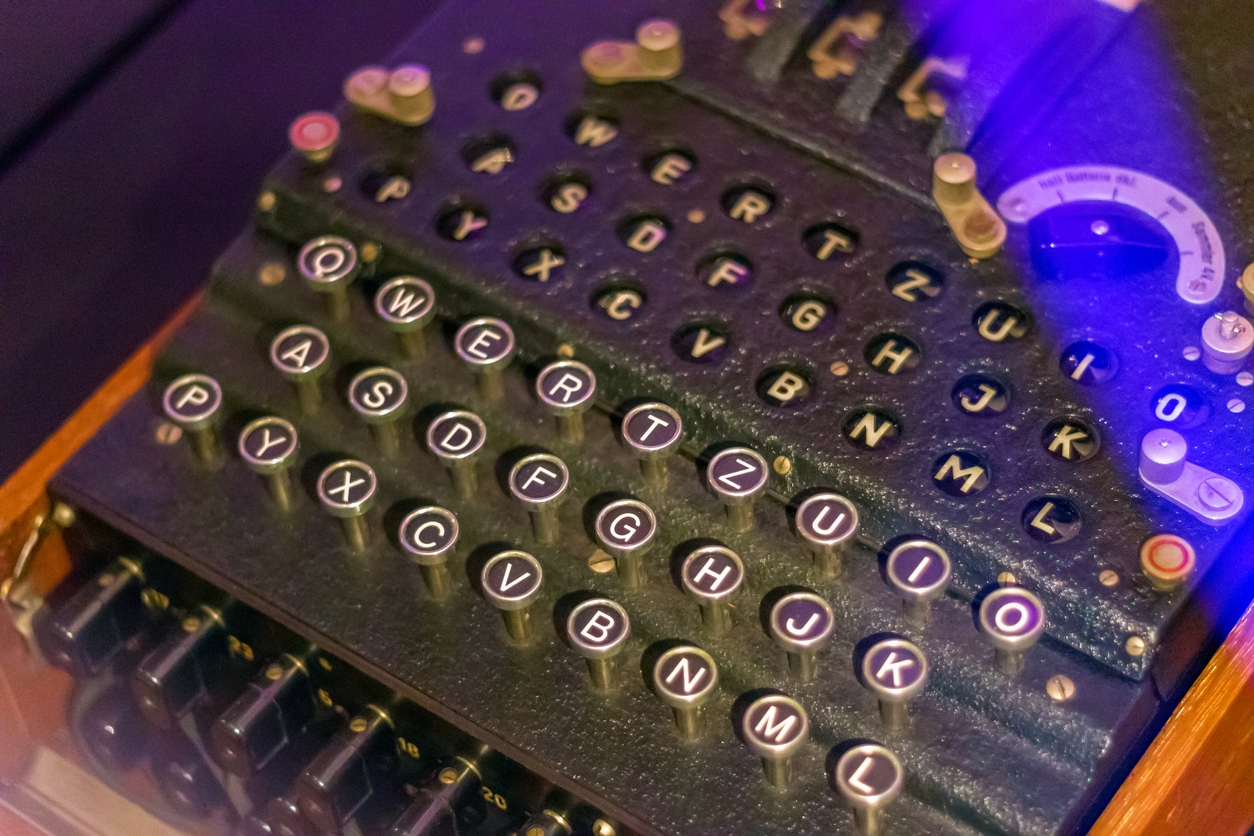
At the outset of World War II, codebreaking was in its infancy, and the challenges were daunting. Military and intelligence agencies grappled with ciphers that ranged from the seemingly inscrutable to the elementary. The use of complex encryption machines, like the German Enigma, added a formidable layer of complexity. The early codebreakers toiled to unravel these intricate systems.
Limitations and Challenges Faced By the Early Codebreakers
In the early days of World War II, codebreakers encountered numerous obstacles. The encryption methods used by the Axis powers, particularly the Germans and the Japanese, were constantly evolving. They employed complex cipher machines and encryption techniques, making it incredibly challenging to intercept and decode their messages. This put immense pressure on Allied cryptanalysts, who had to rely on limited computing technology and often had to break codes by hand.
One notable challenge was the German Enigma machine, which was used to encrypt military communications. While the Enigma code had been broken by the Polish Cipher Bureau before the war, [1] the Germans continued to improve their machines, making it necessary to keep pace with their evolving technology. Early codebreakers faced daunting odds as they sought to crack these sophisticated systems.
Notable Early Successes and Failures
| Effort | Outcome |
| Zimmermann Telegram | Successfully decrypted and influenced the U.S. entry into WWI. |
| Purple Code | US cryptanalysts deciphered Japanese diplomatic messages during WWII. |
| Cryptanalysis of the Enigma | The Allied success in breaking the German Enigma code, impacting the war. |
Despite these challenges, there were significant successes. For example, British codebreakers at Bletchley Park, including Alan Turing, managed to decipher crucial aspects of the Enigma code, providing invaluable intelligence to the Allies. [2] Their work significantly contributed to the success of D-Day and other pivotal operations.
However, there were also setbacks and failures. The Battle of Taranto, for instance, demonstrated the importance of codebreaking.[3] The British Royal Navy executed a successful attack on the Italian fleet at Taranto but failed to protect their own code, leading to significant losses later in the war. These early experiences highlighted the critical role that codebreaking played in the conflict and the need for constant innovation and adaptation in the face of evolving encryption methods.
The Role of Mathematics
An intriguing fact about mathematics is that it played a pivotal role in codebreaking during World War II. It was the foundation upon which codebreakers developed the techniques necessary to decipher encrypted messages and gain a significant advantage in the war.
Mathematical Techniques Used
Mathematics played an important role in deciphering, and interpreting encrypted messages. The two primary mathematical techniques employed were statistical analysis and probability theory.
Statistical Analysis
Codebreakers relied heavily on statistical analysis to identify patterns and anomalies in intercepted messages. [4] This involved counting the frequency of letters, letter combinations, or other elements within the encoded text. Deviations from expected patterns often indicated a breakthrough in decrypting the code. For example, if a particular letter appeared more frequently than others, it might suggest the use of a substitution cipher, a critical clue for decryption.
Probability Theory
Probability theory played a fundamental role in assessing potential solutions and narrowing down the possibilities for decryption. [5] Codebreakers used probabilistic methods to evaluate the likelihood of different encryption keys or settings. This allowed them to prioritize which methods to apply, saving valuable time and resources. By using probability theory, they could focus on the most promising leads, increasing the efficiency of their codebreaking efforts.
Importance of Mathematics in Codebreaking
These mathematical techniques provided a structured and systematic approach to codebreaking, allowing cryptanalysts to decipher complex ciphers used by Axis forces. By applying mathematical rigor to the analysis of intercepted messages, codebreakers increased their chances of breaking enemy codes and gaining critical intelligence during the war. The role of mathematics in this context was not only significant but also instrumental in achieving many key breakthroughs.
The Enigma Machine
The Enigma machine, employed by the Germans during World War II, was a highly complex encryption device that posed a formidable challenge for Allied codebreakers. It consisted of a series of rotors and an intricate wiring system, which scrambled plain text messages into ciphertext, making it exceptionally difficult to decipher. [6]
Breaking the Enigma Code
| Machine/Technology | Description |
| The Bombe | Electro-mechanical device for Enigma decryption. |
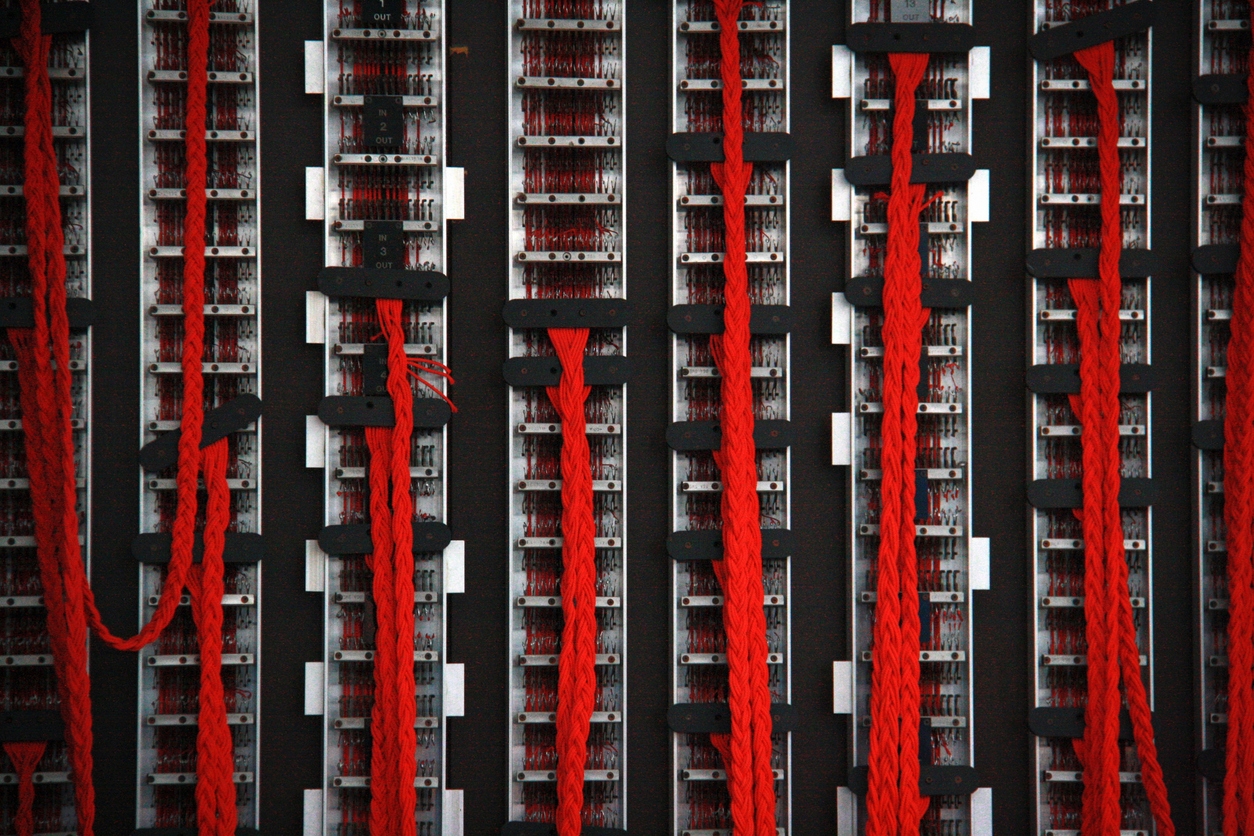
| The Colossus | Early electronic computer used at Bletchley Park. |
| The Navajo Code | The unbroken code used by the Navajo Code Talkers. |
Mathematics played a pivotal role in breaking the Enigma code. The complexity of the machine demanded mathematical analysis to unravel its secrets. One of the key contributions of mathematics was the development of the Bombe machine, an electromechanical device that systematically tested possible Enigma settings.
The Bombe Machine
British mathematician Alan Turing and his team at Bletchley Park developed the Bombe machine. It was a remarkable application of mathematical principles to decode Enigma messages. This electro-mechanical device used mathematical logic to test numerous rotor settings and eliminate improbable combinations, significantly expediting the decryption process.
In essence, mathematics enabled the design and operation of machines like the Bombe, which played a decisive role in cracking the Enigma code. The use of mathematical methods, coupled with the tireless efforts of cryptanalysts, was instrumental in ultimately breaking the Enigma cipher and gaining access to valuable intelligence during the war.
Bletchley Park
Bletchley Park, the British codebreaking center during World War II, served as a hub of innovative thinking and mathematical expertise in the quest to decipher enemy codes. [7] At the heart of this endeavor was the remarkable work of Alan Turing, a brilliant mathematician and computer science pioneer.
| Area of Impact | Contribution |
| Battle of Midway | Breaking Japanese naval codes influenced the outcome. |
| D-Day Invasion | Decrypted German messages assisted the Allied forces. |
| Battle of the Atlantic | Successful U-boat tracking due to codebreaking. |
Alan Turing’s Role
Alan Turing, with his profound understanding of mathematics and logic, played a pivotal role at Bletchley Park. [8] His contributions were instrumental in the development of the Bombe machine, which proved indispensable in decoding Enigma-encrypted messages. Turing’s analytical brilliance allowed him to conceive and implement mathematical principles for tackling the complexities of Enigma.
Turing’s mathematical approach revolutionized codebreaking and had a profound impact on the outcome of World War II. Bletchley Park’s codebreakers, including Alan Turing, demonstrated the indispensable role of mathematics in overcoming the formidable challenges posed by encrypted enemy communications. [9] Their work remains a testament to the power of mathematical thinking in the face of complex problems.
Navajo Code Talkers
During World War II, the Navajo Code Talkers played a crucial and unbreakable role in secure communications. The success of their code was rooted in its exceptional complexity and the indecipherable nature of the Navajo language. [10] Despite the explosion of the written word, the Navajo was an unwritten language.
Unique Code Complexity
The Navajo Code was not merely a substitution cipher, which was often vulnerable to decryption. Instead, it was a complex, multiplexed code, where multiple words or concepts could be represented by a single Navajo word. For instance, the Navajo word “wol-la-chee” meant “ant,” but it also stood for the letter “A” in the English alphabet. Such intricacies made the code incredibly challenging for any potential interceptors to crack.
Unbroken Code
Due to the unique complexity and the untranslatable nature of the Navajo language, the code remained unbroken throughout the war. [11] The intricate system of word substitution and the use of native words made it an insurmountable challenge for enemy codebreakers. The fact that the Navajo Code Talkers played an essential role in secure communications is a testament to the effectiveness of their language’s complexity.
The contributions of Navajo Code Talkers exemplify how complexity, particularly in language, can create an impregnable code, safeguarding vital wartime communications from adversaries. The intricate nature of their code played a pivotal role in maintaining the security and success of Allied operations during the war.
Japanese Codes
In the realm of codebreaking during World War II, the Japanese codes presented a formidable mathematical challenge. These codes demanded complex strategies and relentless efforts from Allied cryptanalysts.
Complex Japanese Codes
The Japanese employed several sophisticated codes during the war, including the Purple code and the JN-25. The Purple code, used for diplomatic communications, was particularly challenging. It was an electromechanical cipher machine that required intricate cryptanalysis to decipher. The JN-25 code, used by the Japanese Navy, involved extensive mathematical analysis and decryption.
Successes in Codebreaking
Despite the complexities, Allied cryptanalysts achieved notable successes in breaking Japanese codes. [12] American codebreakers, such as those at Arlington Hall and the United States Navy’s Communication Special Unit, made significant strides in decrypting Japanese messages. One of the most critical achievements was deciphering the JN-25 naval code, allowing the Allies to gain insights into Japanese naval operations.
These successful codebreaking endeavors were fueled by mathematical rigor, clever analysis, and persistent efforts. Decrypting Japanese codes was no small feat, but the dedication of Allied codebreakers and the mathematical strategies employed played a pivotal role in gaining crucial intelligence during the war.
Impact and Legacy
The impact of successful codebreaking during World War II was profound and far-reaching. The legacy of mathematics in modern cryptography and codebreaking continues to influence intelligence, security, and technology.
World War II Outcomes
Successful codebreaking played a pivotal role in several key aspects of World War II. It provided Allied forces with critical intelligence, enabling them to anticipate enemy movements, intercept secret communications, and gain valuable insights. The deciphering of Enigma and other codes allowed the Allies to strategize effectively, contributing to the outcome of significant battles and missions
Modern Cryptography
| Area of Application | Importance |
| Data Encryption | Securing sensitive information. |
| Internet Security | Protecting online transactions. |
| Digital Signatures | Verifying the authenticity of digital documents. |
The legacy of mathematics in modern cryptography is indisputable. The mathematical techniques and principles developed during World War II laid the foundation for contemporary encryption methods. Today, advanced algorithms, statistical analysis, and probability theory continue to be at the core of cryptographic systems used to secure data, communication, and digital information.
Technological Advancements
Code breaking efforts during the war led to technological advancements in computing and encryption. The development of machines like the Bombe and the Colossus marked the early stages of computer science. These innovations not only revolutionized codebreaking but also set the stage for the development of modern computers.
Conclusion
The mathematical odds of successful codebreaking in World War II were daunting, yet dedicated cryptanalysts, like Alan Turing and the Navajo Code Talkers, rose to the challenge. Their remarkable achievements not only impacted the outcome of the war but also left an enduring legacy in the world of cryptography and technology. The importance of mathematics in safeguarding information and national security remains as vital today as it was during that critical period in history.